La filosofia è scritta in questo grandissimo libro che continuamente ci sta aperto innanzi a gli occhi (io dico l’universo), ma non si può intendere se prima non s’impara a intender la lingua, e conoscer i caratteri, ne’ quali è scritto. Egli è scritto in lingua matematica, e i caratteri son triangoli, cerchi, ed altre figure geometriche, senza i quali mezi è impossibile a intenderne umanamente parola; senza questi è un aggirarsi vanamente per un oscuro laberinto.
― Galileo Galilei, Il Saggiatore (The Assayer)
Joan was quizzical; studied pataphysical science in the home. Late nights all alone with a test tube.
— The Beatles, Maxwell’s Silver Hammer
Unless you’re a member of the Flat Earth Society, I bet you’re pretty confident that the Earth is round. In fact, you’re so confident that you don’t even ask yourself the question why you are so confident. After all, there is overwhelming scientific evidence for the round-Earth hypothesis. There is the old “ships disappearing behind the horizon” proof, there are satellites circling the Earth, there are even photos of the Earth seen from the Moon, the list goes on and on. I picked this particular theory because it seems so obviously true. So if I try to convince you that the Earth is flat, I’ll have to dig very deep into the foundation of your belief systems. Here’s what I’ve found: We believe that the Earth is round not because it’s the truth, but because we are lazy and stingy (or, to give it a more positive spin, efficient and parsimonious). Let me explain…
The New Flat Earth Theory
Let’s begin by stressing how useful the flat-Earth model is in everyday life. I use it all the time. When I want to find the nearest ATM or a gas station, I take out my cell phone and look it up on its flat screen. I’m not carrying a special spherical gadget in my pocket. The screen on my phone is not bulging in the slightest when it’s displaying a map of my surroundings. So, at least within the limits of my city, or even the state, flat-Earth theory works just fine, thank you!
I’d like to make parallels with another widely accepted theory, Einstein’s special relativity. We believe that it’s true, but we never use it in everyday life. The vast majority of objects around us move much slower than the speed of light, so traditional Newtonian mechanics works just fine for us. When was the last time you had to reset your watch after driving from one city to another to account for the effects of time dilation?
The point is that every physical theory is only valid within a certain range of parameters. Physicists have always been looking for the Holy Grail of theories — the theory of everything that would be valid for all values of parameters with no exceptions. They haven’t found one yet.
But, obviously, special relativity is better than Newtonian mechanics because it’s more general. You can derive Newtonian mechanics as a low velocity approximation to special relativity. And, sure enough, the flat-Earth theory is an approximation to the round-Earth theory for small distances. Or, equivalently, it’s the limit as the radius of the Earth goes to infinity.
But suppose that we were prohibited (for instance, by a religion or a government) from ever considering the curvature of the Earth. As explorers travel farther and farther, they discover that the “naive” flat-Earth theory gives incorrect answers. Unlike present-day flat-earthers, who are not scientifically sophisticated, they would actually put some effort to refine their calculations to account for the “anomalies.” For instance, they could postulate that, as you get away from the North Pole, which is the center of the flat Earth, something funny keeps happening to measuring rods. They get elongated when positioned along the parallels (the circles centered at the North Pole). The further away you get from the North Pole, the more they elongate, until at a certain distance they become infinite. Which means that the distances (measured using those measuring rods) along the big circles get smaller and smaller until they shrink to zero.

I know this theory sounds weird at first, but so does special and, even more so, general relativity. In special relativity, weird things happen when your speed is close to the speed of light. Time slows down, distances shrink in the direction of flight (but not perpendicular to it!), and masses increase. In general relativity, similar things happen when you get closer to a black hole’s event horizon. In both theories things diverge as you hit the limit — the speed of light, or the event horizon, respectively.
Back to flat Earth — our explorers conquer space. They have to extend their weird geometry to three dimensions. They find out that horizontally positioned measuring rods shrink as you go higher (they un-shrink when you point them vertically). The intrepid explorers also dig into the ground, and probe the depths with seismographs. They find another singularity at a particular depth, where the horizontal dilation of measuring rods reaches infinity (round-Earthers call this the center of the Earth).
This generalized flat-Earth theory actually works. I know that, because I have just described the spherical coordinate system. We use it when we talk about degrees of longitude and latitude. We just never think of measuring distances using spherical coordinates — it’s too much work, and we are lazy. But it’s possible to express the metric tensor in those coordinates. It’s not constant — it varies with position — and it’s not isotropic — distances vary with direction. In fact, because of that, flat Earthers would be better equipped to understand general relativity than we are.
So is the Earth flat or spherical? Actually it’s neither. Both theories are just approximations. In cartesian coordinates, the Earth is the shape of a flattened ellipsoid, but as you increase the resolution, you discover more and more anomalies (we call them mountains, canyons, etc.). In spherical coordinates, the Earth is flat, but again, only approximately. The biggest difference is that the math is harder in spherical coordinates.
Have I confused you enough? On one level, unless you’re an astronaut, your senses tell you that the Earth is flat. On the other level, unless you’re a conspiracy theorist who believes that NASA is involved in a scam of enormous proportions, you believe that the Earth is pretty much spherical. Now I’m telling you that there is a perfectly consistent mathematical model in which the Earth is flat. It’s not a cult, it’s science! So why do you feel that the round Earth theory is closer to the truth?
The Occam’s Razor
The round Earth theory is just simpler. And for some reason we cling to the belief that nature abhors complexity (I know, isn’t it crazy?). We even express this belief as a principle called the Occam’s razor. In a nutshell, it says that:
Among competing hypotheses, the one with the fewest assumptions should be selected.
Notice that this is not a law of nature. It’s not even scientific: there is no way to falsify it. You can argue for the Occam’s razor on the grounds of theology (William of Ockham was a Franciscan friar) or esthetics (we like elegant theories), but ultimately it boils down to pragmatism: A simpler theory is easier to understand and use.

It’s a mistake to think that Occam’s razor tells us anything about the nature of things, whatever that means. It simply describes the limitations of our mind. It’s not nature that abhors complexity — it’s our brains that prefer simplicity.
Unless you believe that physical laws have an independent existence of their own.
The Layered Cake Hypothesis
Scientists since Galileo have a picture of the Universe that consists of three layers. The top layer is nature that we observe and interact with. Below are laws of physics — the mechanisms that drive nature and make it predictable. Still below is mathematics — the language of physics (that’s what Galileo’s quote at the top of this post is about). According to this view, physics and mathematics are the hidden components of the Universe. They are the invisible cogwheels and pulleys whose existence we can only deduce indirectly. According to this view, we discover the laws of physics. We also discover mathematics.
Notice that this is very different from art. We don’t say that Beethoven discovered the Fifth Symphony (although Igor Stravinsky called it “inevitable”) or that Leonardo da Vinci discovered the Mona Lisa. The difference is that, had not Beethoven composed his symphony, nobody would; but if Cardano hadn’t discovered complex numbers, somebody else probably would. In fact there were many cases of the same mathematical idea being discovered independently by more than one person. Does this prove that mathematical ideas exist the same way as, say, the moons of Jupiter?
Physical discoveries have a very different character than mathematical discoveries. Laws of physics are testable against physical reality. We perform experiments in the real world and if the results contradict a theory, we discard the theory. A mathematical theory, on the other hand, can only be tested against itself. We discard a theory when it leads to internal contradictions.
The belief that mathematics is discovered rather than invented has its roots in Platonism. When we say that the Earth is spherical, we are talking about the idea of a sphere. According to Plato, these ideas do exist independently of the observer — in this case, a mathematician who studies them. Most mathematicians are Platonists, whether they admit it or not.
Being able to formulate laws of physics in terms of simple mathematical equations is a thing of beauty and elegance. But you have to realize that history of physics is littered with carcasses of elegant theories. There was a very elegant theory, which postulated that all matter was made of just four elements: fire, air, water, and earth. The firmament was a collection of celestial spheres (spheres are so Platonic). Then the orbits of planets were supposed to be perfect circles — they weren’t. They aren’t even elliptical, if you study them close enough.
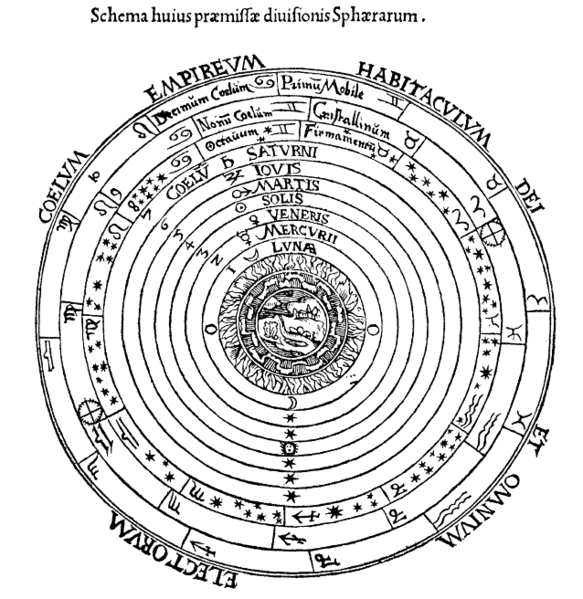
Celestial spheres. An elegant theory, slightly complicated by the need to introduce epicycles to describe the movements of planets
The Impasse
But maybe at the level of elementary particles and quantum fields some of this presumed elegance of the Universe shines through? Well, not really. If the Universe obeyed the Occam’s razor, it would have stopped at two quarks, up and down. Nobody needs the strange and the charmed quarks, not to mention the bottom and the top quarks. The Standard Model of particle physics looks like a kitchen sink filled with dirty dishes. And then there is gravity that resists all attempts at grand unification. Strings were supposed to help but they turned out to be as messy as the rest of it.
Of course the current state of impasse in physics might be temporary. After all we’ve been making tremendous progress up until about the second half of the twentieth century (the most recent major theoretical breakthroughs were the discovery of the Higgs mechanism in 1964 and the proof or renormalizability of the Standard Model in 1971).
On the other hand, it’s possible that we might be reaching the limits of human capacity to understand the Universe. After all, there is no reason to believe that the structure of the Universe is simple enough for the human brain to analyze. There is no guarantee that it can be translated into the language of physics and mathematics.
Is the Universe Knowable?
In fact, if you think about it, our expectation that the Universe is knowable is quite arbitrary. On the one hand you have the vast complex Universe, on the other hand you have slightly evolved monkey brains that have only recently figured out how to use tools and communicate using speech. The idea that these brains could produce and store a model of the Universe is preposterous. Granted, our monkey brains are a product of evolution, and our survival depends on those brains being able to come up with workable models of our environment. These models, however, do not include the microcosm or the macrocosm — just the narrow band of phenomena in between. Our senses can perceive space and time scales within about 8 orders of magnitude. For comparison, the Universe is about 40 orders of magnitude larger than the size of the atomic nucleus (not to mention another 20 orders of magnitude down to Planck length).
The evolution came up with an ingenious scheme to deal with the complexities of our environment. Since it is impossible to store all information about the Universe in the very limited amount of memory at our disposal, and it’s impossible to run the simulation in real time, we have settled for the next best thing: creating simplified partial models that are composable.
The idea is that, in order to predict the trajectory of a spear thrown at a mammoth, it’s enough to roughly estimate the influence of a constant downward pull of gravity and the atmospheric drag on the idealized projectile. It is perfectly safe to ignore a lot of subtle effects: the non-uniformity of the gravitational field, air-density fluctuations, imperfections of the spear, not to mention relativistic effects or quantum corrections.
And this is the key to understanding our strategy: we build a simple model and then calculate corrections to it. The idea is that corrections are small enough as not to destroy the premise of the model.
Celestial Mechanics
A great example of this is celestial mechanics. To the lowest approximation, the planets revolve around the Sun along elliptical orbits. The ellipse is a solution of the one body problem in a central gravitational field of the Sun; or a two body problem, if you also take into account the tiny orbit of the Sun. But planets also interact with each other — in particular the heaviest one, Jupiter, influences the orbits of other planets. We can treat these interactions as corrections to the original solution. The more corrections we add, the better predictions we can make. Astronomers came up with some ingenious numerical methods to make such calculations possible. And yet it’s known that, in the long run, this procedure fails miserably. That’s because even the tiniest of corrections may lead to a complete change of behavior in the far future. This is the property of chaotic systems, our Solar System being just one example of such. You must have heard of the butterfly effect — the Universe is filled with this kind of butterflies.
Ephemerides: Tables showing positions of planets on the firmament.
The Microcosm
Anyone who is not shocked by quantum
theory has not understood a single word.— Niels Bohr
At the other end of the spectrum we have atoms and elementary particles. We call them particles because, to the lowest approximation, they behave like particles. You might have seen traces made by particles in a bubble chamber.
Elementary particles might, at first sight, exhibit some properties of macroscopic objects. They follow paths through the bubble chamber. A rock thrown in the air also follows a path — so elementary particles can’t be much different from little rocks. This kind of thinking led to the first model of the atom as a miniature planetary system. As it turned out, elementary particles are nothing like little rocks. So maybe they are like waves on a lake? But waves are continuous and particles can be counted by Geiger counters. We would like elementary particles to either behave like particles or like waves but, despite our best efforts, they refuse to nicely fall into one of the categories.
There is a good reason why we favor particle and wave explanations: they are composable. A two-particle system is a composition of two one-particle systems. A complex wave can be decomposed into a superposition of simpler waves. A quantum system is neither. We might try to separate a two-particle system into its individual constituents, but then we have to introduce spooky action at a distance to explain quantum entanglement. A quantum system is an alien entity that does not fit our preconceived notions, and the main characteristic that distinguishes it from classical phenomena is that it’s not composable. If quantum phenomena were composable in some other way, different from particles or waves, we could probably internalize it. But non-composable phenomena are totally alien to our way of thinking. You might think that physicists have some deeper insight into quantum mechanics, but they don’t. Richard Feynman, who was a no-nonsense physicist, famously said, “If you think you understand quantum mechanics, you don’t understand quantum mechanics.” The problem with understanding quantum mechanics is not that it’s too complex. The problem is that our brains can only deal with concepts that are composable.
It’s interesting to notice that by accepting quantum mechanics we gave up on composability on one level in order to decompose something at another level. The periodic table of elements was the big challenge at the beginning of the 20th century. We already knew that earth, water, air, and fire were not enough. We understood that chemical compounds were combinations of atoms; but there were just too many kinds of atoms, and they could be grouped into families that shared similar properties. Atom was supposed to be indivisible (the Greek word ἄτομος [átomos] means indivisible), but we could not explain the periodic table without assuming that there was some underlying structure. And indeed, there is structure there, but the way the nucleus and the electrons compose in order to form an atom is far from trivial. Electrons are not like planets orbiting the nucleus. They form shells and orbitals. We had to wait for quantum mechanics and the Fermi exclusion principle to describe the structure of an atom.
Every time we explain one level of complexity by decomposing it in terms of simpler constituents we seem to trade off some of the simplicity of the composition itself. This happened again in the sixties, when physicists were faced with a confusing zoo of elementary particles. It seemed like there were hundreds of strongly interacting particles, hadrons, and every year was bringing new discoveries. This mess was finally cleaned up by the introduction of quarks. It was possible to categorize all hadrons as composed of just six types of quarks. This simplification didn’t come without a price, though. When we say an atom is composed of the nucleus and electrons, we can prove it by knocking off a few electrons and studying them as independent particles. We can even split the nucleus into protons and neutrons, although the neutrons outside of a nucleus are short lived. But no matter how hard we try, we cannot split a proton into its constituent quarks. In fact we know that quarks cannot exist outside of hadrons. This is called quark- or color-confinement. Quarks are supposed to come in three “colors,” but the only composites we can observe are colorless. We have stretched the idea of composition by accepting the fact that a composite structure can never be decomposed into its constituents.
I’m Slightly Perturbed
How do physicists deal with quantum mechanics? They use mathematics. Richard Feynman came up with ingenious ways to perform calculations in quantum electrodynamics using perturbation theory. The idea of perturbation theory is that you start with the simple approximation and keep adding corrections to it, just like with celestial mechanics. The terms in the expansion can be visualized as Feynman diagrams. For instance, the lowest term in the interaction between two electrons corresponds to a diagram in which the electrons exchange a virtual photon.

This terms gives the classical repulsive force between two charged particles. The first quantum correction to it involves the exchange of two virtual photons. And here’s the kicker: this correction is not only larger than the original term — it’s infinite! So much for small corrections. Yes, there are tricks to shove this infinity under the carpet, but everybody who’s not fooling themselves understands that the so called renormalization is an ugly hack. We don’t understand what the world looks like at very small scales and we try to ignore it using tricks that make mathematicians faint.
Physicists are very pragmatic. As long as there is a recipe for obtaining results that can be compared with the experiment, they are happy with a theory. In this respect, the Standard Model is the most successful theory in the Universe. It’s a unified quantum field theory of electromagnetism, strong, and weak interactions that produces results that are in perfect agreement with all high-energy experiments we were able to perform to this day. Unfortunately, the Standard Model does not give us the understanding of what’s happening. It’s as if physicists were given an alien cell phone and figured out how to use various applications on it but have no idea about the internal workings of the gadget. And that’s even before we try to involve gravity in the model.
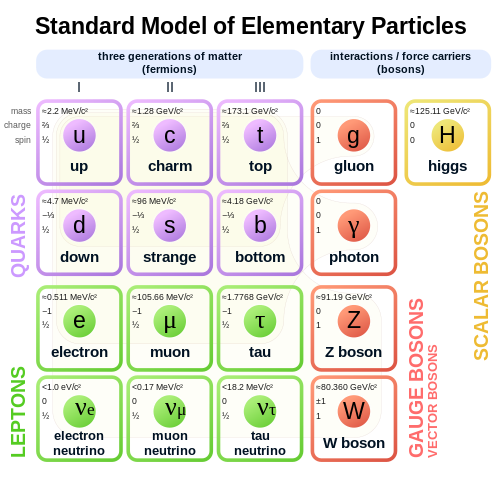
The “periodic table” of elementary particles.
The prevailing wisdom is that these are just little setbacks on the way toward the ultimate theory of everything. We just have to figure out the correct math. It may take us twenty years, or two hundred years, but we’ll get there. The hope that math is the answer led theoretical physicists to study more and more esoteric corners of mathematics and to contribute to its development. One of the most prominent theoretical physicists, Edward Witten, the father of M-theory that unified a number of string theories, was awarded the prestigious Fields Medal for his contribution to mathematics (Nobel prizes are only awarded when a theory is confirmed by experiment which, in the case of string theory, may be a be long way off, if ever).
Math is About Composition
If mathematics is discoverable, then we might indeed be able to find the right combination of math and physics to unlock the secrets of the Universe. That would be extremely lucky, though.
There is one property of all of mathematics that is really striking, and it’s most clearly visible in foundational theories, such as logic, category theory, and lambda calculus. All these theories are about composability. They all describe how to construct more complex things from simpler elements. Logic is about combining simple predicates using conjunctions, disjunctions, and implications. Category theory starts by defining a composition of arrows. It then introduces ways of combining objects using products, coproducts, and exponentials. Typed lambda calculus, the foundation of computer languages, shows us how to define new types using product types, sum types, and functions. In fact it can be shown that constructive logic, cartesian closed categories, and typed lambda calculus are three different formulations of the same theory. This is known as the Curry Howard Lambek isomorphism. We’ve been discovering the same thing over and over again.
It turns out that most mathematical theories have a skeleton that can be captured by category theory. This should not be a surprise considering how the biggest revolutions in mathematics were the result of realization that two or more disciplines were closely related to each other. The latest such breakthrough was the proof of the Fermat’s last theorem. This proof was based on the Taniyama-Shimura conjecture that related the study of elliptic curves to modular forms — two radically different branches of mathematics.
Earlier, geometry was turned upside down when it became obvious that one can define shapes using algebraic equations in cartesian coordinates. This retooling of geometry turned out to be very advantageous, because algebra has better compositional qualities than Euclidean-style geometry.
Finally, any mathematical theory starts with a set of axioms, which are combined using proof systems to produce theorems. Proof systems are compositional which, again, supports the view that mathematics is all about composition. But even there we hit a snag when we tried to decompose the space of all statements into true and false. Gödel has shown that, in any non-trivial theory, we can formulate a statement that can neither be proved to be right or wrong, and thus the Hilbert’s great project of defining one grand mathematical theory fell apart. It’s as if we have discovered that the Lego blocks we were playing with were not part of a giant Lego spaceship.
Where Does Composability Come From?
It’s possible that composability is the fundamental property of the Universe, which would make it comprehensible to us humans, and it would validate our physics and mathematics. Personally, I’m very reluctant to accept this point of view, because it would give intelligent life a special place in the grand scheme of things. It’s as if the laws of the Universe were created in such a way as to be accessible to the brains of the evolved monkeys that we are.
It’s much more likely that mathematics describes the ways our brains are capable of composing simpler things into more complex systems. Anything that we can comprehend using our brains must, by necessity, be decomposable — and there are only so many ways of putting things together. Discovering mathematics means discovering the structure of our brains. Platonic ideals exist only as patterns of connections between neurons.
The amazing scientific progress that humanity has been able to make to this day was possible because there were so many decomposable phenomena available to us. Granted, as we progressed, we had to come up with more elaborate composition schemes. We have discovered differential equations, Hilbert spaces, path integrals, Lie groups, tensor calculus, fiber bundles, etc. With the combination of physics and mathematics we have tapped into a gold vein of composable phenomena. But research takes more and more resources as we progress, and it’s possible that we have reached the bedrock that may be resistant to our tools.
We have to seriously consider the possibility that there is a major incompatibility between the complexity of the Universe and the simplicity of our brains. We are not without recourse, though. We have at our disposal tools that multiply the power of our brains. The first such tool is language, which helps us combine brain powers of large groups of people. The invention of the printing press and then the internet helped us record and gain access to vast stores of information that’s been gathered by the combined forces of teams of researchers over long periods of time. But even though this is quantitative improvement, the processing of this information still relies on composition because it has to be presented to human brains. The fact that work can be divided among members of larger teams is proof of its decomposability. This is also why we sometimes need a genius to make a major breakthrough, when a task cannot be easily decomposed into smaller, easier, subtasks. But even genius has to start somewhere, and the ability to stand on the shoulders of giants is predicated on decomposability.
Can Computers Help?
The role of computers in doing science is steadily increasing. To begin with, once we have a scientific theory, we can write computer programs to perform calculations. Nobody calculates the orbits of planets by hand any more — computers can do it much faster and error free. We are also beginning to use computers to prove mathematical theorems. The four-color problem is an example of a proof that would be impossible without the help of computers. It was decomposable, but the number of special cases was well over a thousand (it was later reduced to 633 — still too many, even for a dedicated team of graduate students).
Every planar map can be colored using only four colors.
Computer programs that are used in theorem proving provide a level of indirection between the mind of a scientist and formal manipulations necessary to prove a theorem. A programmer is still in control, and the problem is decomposable, but the number of components may be much larger, often too large for a human to go over one by one. The combined forces of humans and computers can stretch the limits of composability.
But how can we tackle problems that cannot be decomposed? First, let’s observe that in real life we rarely bother to go through the process of detailed analysis. In fact the survival of our ancestors depended on the ability to react quickly to changing circumstances, to make instantaneous decisions. When you see a tiger, you don’t decompose the image into individual parts, analyze them, and put together a model of a tiger. Image recognition is one of these areas where the analytic approach fails miserably. People tried to write programs that would recognize faces using separate subroutines to detect eyes, noses, lips, ears, etc., and composing them together, but they failed. And yet we instinctively recognize faces of familiar people at a glance.
Neural Networks and the AI
We are now able to teach computers to classify images and recognize faces. We do it not by designing dedicated algorithms; we do it by training artificial neural networks. A neural network doesn’t start with a subsystem for recognizing eyes or noses. It’s possible that, in the process of training, it will develop the notions of lines, shadows, maybe even eyes and noses. But by no means is this necessary. Those abstractions, if they evolve, would be encoded in the connections between its neurons. We might even help the AI develop some general abstractions by tweaking its architecture. It’s common, for instance, to include convolutional layers to pre-process the input. Such a layer can be taught to recognize local features and compress the input to a more manageable size. This is very similar to how our own vision works: the retina in our eye does this kind of pre-processing before sending compressed signals through the optic nerve.

Compression is the key to matching the complexity of the task at hand to the simplicity of the system that is processing it. Just like our sensory organs and brains compress the inputs, so do neural networks. There are two kinds of compression: the kind that doesn’t lose any information, just removing the redundancy in the original signal; and the lossy kind that throws away irrelevant information. The task of deciding what information is irrelevant is in itself a process of discovery. The difference between the Earth and a sphere is the size of the Himalayas, but we ignore it when when we look at the globe. When calculating orbits around the Sun, we shrink all planets to points. That’s compression by elimination of details that we deem less important for the problem we are solving. In science, this kind of compression is called abstraction.
We are still way ahead of neural networks in our capacity to create abstractions. But it’s possible that, at some point, they’ll catch up with us. The problem is: Will we be able to understand machine-generated abstractions? We are already at the limits of understanding human-generated abstractions. You may count yourself a member of a very small club if you understand the statement “monad is a monoid in the category of endofunctors” that is chock full of mathematical abstractions. If neural networks come up with new abstractions/compression schemes, we might not be able to reverse engineer them. Unlike a human scientist, an AI is unlikely to be able to explain to us how it came up with a particular abstraction.
I’m not scared about a future AI trying to eliminate human kind (unless that’s what its design goals are). I’m afraid of the scenario in which we ask the AI a question like, “Can quantum mechanics be unified with gravity?” and it will answer, “Yes, but I can’t explain it to you, because you don’t have the brain capacity to understand the explanation.”
And this is the optimistic scenario. It assumes that such questions can be answered within the decomposition/re-composition framework. That the Universe can be decomposed into particles, waves, fields, strings, branes, and maybe some new abstractions that we haven’t even though about. We would at least get the satisfaction that we were on the right path but that the number of moving parts was simply too large for us to assimilate — just like with the proof of the four-color theorem.
But it’s possible that this reductionist scenario has its limits. That the complexity of the Universe is, at some level, irreducible and cannot be captured by human brains or even the most sophisticated AIs.
There are people who believe that we live in a computer simulation. But if the Universe is irreducible, it would mean that the smallest computer on which such a simulation could be run is the Universe itself, in which case it doesn’t make sense to call it a simulation.
Conclusion
The scientific method has been tremendously successful in explaining the workings of our world. It led to exponential expansion of science and technology that started in the 19th century and continues to this day. We are so used to its successes that we are betting the future of humanity on it. Usually when somebody attacks the scientific method, they are coming from the background of obscurantism. Such attacks are easily rebuffed or dismissed. What I’m arguing is that science is not a property of the Universe, but rather a construct of our limited brains. We have developed some very sophisticated tools to create models of the Universe based on the principle of composition. Mathematics is the study of various ways of composing things and physics is applied composition. There is no guarantee, however, that the Universe is decomposable. Assuming that would be tantamount to postulating that its structure revolves around human brains, just like we used to believe that the Universe revolves around Earth.
You can also watch my talk on this subject.

January 11, 2018 at 9:39 am
Typed lambda calculus is the foundation of some programming languages; certainly not all of them.
In the particular case of what you call the Curry Howard Lambek isomorphism, this was not so much a re-discovery of the same thing, as much as several disciplines that split from a single core where the pertinent properties already lay (Aristotelian-style logic was studied as an algebra in the 19th century, and much of abstract algebra was built through the generalization of the algebraic logic. Frege then proposed an alternative language to study Aristotelian-style logic — to the consternation of the algebraists, which though that one language was enough; Church’s lambda calculus began as an attempt to simplify Frege’s calculus). The shared properties already exist in the underlying “studied object” which is Aristotelian-style logic. That particular isomorphism no longer works (at least not in the same way) when other languages or underlying logics are considered.
January 11, 2018 at 12:29 pm
Aside from the ironic title, very nice and deep article.
It’s odd for me to start reading Italian lines at the beginning of your post.
The curvature of the spacetime for GR is not as easy as the spherical earth for the distance metrics, even tough the analogy would be suggestive. With your words, our monkey brains, deluded by physics and math, should rather study (the much more helpful) medicine.
Ok, I’m writing this comment really because I liked your doubts about reductionism (I often discussed about the true meaning of emergence) and I have only a math question for you:
what do you think about the Bell inequality? How to deeply understand it without the superficial pragmatism of many physicists?
I know, I’ve already asked you similar quantum questions in the past 😉
January 11, 2018 at 1:18 pm
This whole mess with the Copenhagen interpretation, spooky action at a distance, and Bell inequalities is a perfect example of failure of decomposition. First, we insist on decomposing the experiment into the quantum part and the classical observer. This forces us into wave function collapse or many-world interpretation. Then we insist that a two-particle state must be a composition of one-particle states. We construct an experiment based on this assumption, and we find out that observations of so constructed one-particle states are strangely correlated. So we try to explain it using hidden variables, again decomposing the state of a particle into visible and hidden state. Bell inequalities describe the properties of this decomposition. Experimental results show that these inequalities are violated, which means that our attempts at decomposition have failed.
January 11, 2018 at 11:54 pm
Sorry, mine was not only a math question, I intended to ask you the physical interpretation, in your opinion. The pure math sounds very easy: the tensor product state is factorizable (decomposable in your words) if the determinant of the coefficients is non-zero, otherwise it is an entangled state.
Now, your suggestive title and the first part of your article seems to suggest that there can be a simpler and more elegant explanation for apparently complex like a spooky action at a distance.
Quoting your example, the “ptolemaic” (sorry for the rough metaphor) model
“The further away you get from the North Pole, the more they elongate, until at a certain distance they become infinite. Which means that the distances (measured using those measuring rods) along the big circles get smaller and smaller until they shrink to zero.”
can be better explained by the cooler “copernican” truth: the south pole is a unique point in the space and the earth is a sphere (you can’t deny the beauty of the truth).
What’s the equivalent solution for the spooky action? A wormhole in another dimension? A conspiracy theory about experimentalists hiding some measurement bias? lol the latter looks more credible
January 12, 2018 at 2:57 am
You say “the Earth is a sphere,” call it the “truth,” and use the esthetic argument of beauty.
Well, it’s not a sphere. Can we agree on that before we start the discussion?
January 12, 2018 at 3:02 am
Of course there are mountains and it is not a perfect sphere. And you can replace the esthetic argument of beauty with your favorite criteria. Sure, please go on.
January 12, 2018 at 3:13 am
What about the “truth” thingie?
January 12, 2018 at 3:23 am
If I had a “simpler, more elegant” explanation, I would be contradicting myself. I can’t deny that there is a slight possibility that such an explanation exists (Gerard ‘t Hooft seems to be trying that), but I’m suggesting that maybe there is no simple explanation. That the task of translating the Universe into simple elegant ideas that we love so much might be impossible. Just like we now know, thanks to Goedel, that building the one and only true axiomatic theory of all of math is impossible.
January 12, 2018 at 3:26 am
Do you want to say that you are able to build a mathematically equivalent physics where the earth is flat by means of a coordinate transformation? Do you really want to try to rewrite all the physics laws accordingly? Looks pretty impossible in practice
January 12, 2018 at 3:29 am
Ok I understand your answer. Perfect. Thank you very much
January 12, 2018 at 4:00 am
In more technicall words, the general coordinate transformation you mention (when you try to describe the flat earth model in a more sophisticated way) maybe refers to generally covariant theories (GR ), but unfortunately quantum mechanics it is not, so basically I don’t think that the Bell inequality can be explained by any coordinate transformation. I don’t even think that you can achieve a flat earth description by means of a Lorentz transformation.
But, generally speaking, I understand the meaning of your article, I like it and I’ve appreciated very much your kind replies. Thank you again for accepting my comments.
January 12, 2018 at 5:19 am
Actually, Einstein did it for me. Would it be awkward? You bet! But not impossible. One thing is for sure: neither is more “true” than the other. More convenient, more practical, maybe even elegant, pleasing, yes. But “true”?
January 12, 2018 at 6:14 am
It is not a Lorentz transformation when flat earth fans say that geodesics are straight lines while we know that the earth mass curves them! Of course you would propose some quite strange geodesics but first of all… Distance yourself as much as possible from those completely wrong claims of obscurantism. 😉
Notice that the presence of an indecidable statements is a much weaker limit for a theory than the current state of the art of quantum physics: the entanglement is an example in which you can’t even rely on logical counterfactual definiteness…
January 12, 2018 at 6:45 am
Are you aware of “radical constructivism”? It is an epistemic theory in which it is explored how we, as self organised systems, can know what we know. It’s a bit out of fashion and was developed at the beginnings of cybernetics by von Foerster, Maturana & Varela, von Glasersfeld and others. It is rooted in biology (Maturana) and was transferred to other disciplines such as social sciences (Luhmann).
A precursor of radical constructivism was developed by Piaget (dubbed genetic epistemology). He investigated how logical-mathematical structures develop in children. Interestingly he formulated a category theoretic theory of cognitive development [1].
[1] https://www.karger.com/Article/Abstract/275811
January 12, 2018 at 6:55 am
I was talking about expressing the metric tensor in curvilinear coordinates.
January 12, 2018 at 6:55 am
This article is not only an uneducated embarrassment to modern scientific progress, but also a dangerous attempt to spread misinformation by somebody who is simply too lazy and/or incapable to understand and accept the scientific and mathematical facts of the matter. The only real achievement here is that you managed to write so much content without really saying anything of value.
To summarize: You, sir, are an idiot, as is any person who unironically believes in any flat earth theory. Educate yourself, and stop spreading lies in an attempt to feel important. You’re not.
Feel free to reply to this comment and try to deflect or argue, or maybe even take the “Thanks so much for your feedback!” approach so that you can feel like the good guy in the situation. In the end, though, the simple fact is that you are wrong, and that’s all there is to it.
January 12, 2018 at 5:42 pm
The person posting as “Read A. Book” did not read the article. Obviously they saw the title and skimmed maybe 4 lines at most and decided to shit through their keyboard. Ironic.
January 13, 2018 at 3:36 am
I don’t understand what is your conclusion, when you apply the metric tensor in curvilinear coordinates.
What we can read in GR books is that
1) there is no topological map from R3 to S2, but we must restrict to open regions and
2) e.g. for application in cosmology the transformation r=sinθ starting a the North Pole have a coordinate singularity at the equator, etc.. etc…
Eventually I fail to see what the above has to do with the absurd flat earth model (if not little or nothing). Do you have an extended blog/post/arxiv/link where the calculation you mention is being performed and the results you mean are clearly stated?
January 13, 2018 at 4:10 am
Sure, there is a singularity at θ=π. Nothing little renormalization can’t fix, though. In the world I’m describing, there is probably the first scientist who courageously walked through the south pole and survived it. He’s a hero there.
January 13, 2018 at 5:01 am
Why are you mentioning renormalization? Coordinate singularities depend on the choice of coordinates and they go away only if one uses more well-behaved coordinates, but otherwise they can never go away, they can be useful instead like the Schwarzschild coordinates for black holes: so do you allude to the Schwarzschild metric and the event horizon? Finally, if you don’t want to provide a link to a detailed calculation or some in-depth analysis, it is fine, ok, thanks anyway.
January 14, 2018 at 6:14 pm
Excellent article! Reminds me of ‘Zen and Art of Motorcycle maintenance’ in a way.
‘There are people who believe that we live in a computer simulation. But if the Universe is irreducible, it would mean that the smallest computer on which such a simulation could be run is the Universe itself, in which case it doesn’t make sense to call it a simulation.’ -> Not necessarily. Maybe the first sentence should be corrected to: ‘There are people who believe that they live in a computer simulation’. For such folks, there is no “we” – that is part of the simulation. There is no need for a hypothetical super agent to simulate the entire universe, only the information that the single individual receives.
So it is a choice that each of us have – to either believe that I am in a simulation construct or that I’m in a “real” world.
‘There is no guarantee, however, that the Universe is decomposable’ -> Indeed. But there is no meaningful alternative assumption we can make?
January 21, 2018 at 10:56 am
‘Irreducible’ again means ‘irreducible our way’, we again talk about our limitation rather than about the universe itself.
The way we reduce and model our environment may not be tied to the specific setup of the neural network each of us is, it may be tied to the fundamental principles neural networks operate: http://colah.github.io/posts/2015-09-NN-Types-FP/
The first generations of smart machines, although able to handle models with much more moving parts, may not be smarter than us on the fundamental level, and could fail to understand the universe in exactly the same way we do.
January 25, 2018 at 4:29 am
“The four-color problem is an example of a proof that would be impossible without the help of computers.” Is there a proof of that? Is there a category proof of it (iow, what would an equivalent proof be)?
My interest is in using Category Theory in Linguistics, so the next question in that context would be: Would the word “help” be a morphism?
Just getting into the fascinating world of category theory, so please excuse any stupid questions or statements.
January 27, 2018 at 7:55 am
Does this not consequently open up an important path of inquiry which looks more deeply into this construct and it’s construction? If compositionality is an essential feature of this construct or it’s construction, then is it it’s only feature? A main feature? More broadly, doesn’t this lead to a necessary investigation in the nature, not just of our brain, but in the phenomenology of consciousness?
Thanks so much for your blog and raising such thought provoking questions within the community of software engineers. It’s truly inspiring and motivating.
Very best,
Jun
March 8, 2019 at 10:49 am
I really enjoyed this article, it blew me away and has given me more to think about then any article in recent memory has.
However… we aren’t evolved monkeys. I know you understand we aren’t literally evolved from monkeys, but I do think that we are inviting in a specific belief system when we let ourselves casually refer to ourselves as evolved monkeys. That specific belief system is dangerous and we can avoid ever letting it take root by not letting us slip into this generalization.
We share common ancestors with monkeys and are both evolved from these common ancestors. To say that we are evolved monkeys suggests that to be more intelligent, or more human like is to be more evolved. It imposes a human set of values upon evolution which inherently has no such of values.
Douglas Adams puts it best
“For instance, on the planet Earth, man had always assumed that he was more intelligent than dolphins because he had achieved so much—the wheel, New York, wars and so on—whilst all the dolphins had ever done was muck about in the water having a good time. But conversely, the dolphins had always believed that they were far more intelligent than man—for precisely the same reasons.”
😛
March 8, 2019 at 11:15 am
Believe it or not, I actually thought about this, and used the word “evolved” deliberately; mind you, not “better” or “smarter,” but “evolved.” There is a directionality in evolution: life develops toward systems that are better at creating entropy. It’s well known that a forest is a better transformer of solar energy to entropy than, say, a steppe, or a desert. Human civilization is, hands down, the most evolved entropy generator. Dolphins don’t even come close.
November 1, 2021 at 12:48 pm
Wow!!!
March 10, 2022 at 8:27 am
I don’t think non-decomposable systems are at all incomprehensible to humans, they surround us and we participate in them all the time, make them, we are made of them, we are them. I think they’re just incomprehensible to reductionist or universalizing mathemarical programs. The arts are full of them — the tension or resonance within a painting, a beat in a stage play, the interplay of overlapping rhythms in a song. (Precisely what you lose when you apply a Fourier transform.)
I’ve been reading a lot of Deleuze recently, and he gives an excellent accounting of non-decomposable systems; the chapter “1914: One or Several Wolves?” of A Thousand Plateaus comes to mind. Unfortunately the insights he provides are not decomposable. You sort of just have to let the nonsense words wash over you and eventually something clicks. Rather like a Zen Koan.
My private suspicion is that the “abstract purity” aspired to by mathematicians and physicists (each cult in their own fashion) — the distrust of the senses, the faith that they can discover eternal, immutable truths, that everything must stem from a simple set of generators and rules — is precisely what prevents them from finding quantum theory intuitive. Unfortunately, the people who could explain it to them are kept out of the subject by the thick barrier of rigorous purity that all entrants are expected to climb. So the cults grow contorted and withered, trapped inside the formalist walls they themselves built.
October 2, 2022 at 6:57 pm
I just want one of you guys to post your full mathematical model. Not the conspiracy theories. The pure math proving your position.
December 15, 2022 at 10:11 pm
Using your flat map works just fine. Does it show the mountains hills and valleys? Even on this human scale the earth is not flat.